connect
Block diagram interconnections of dynamic systems
Syntax
Description
sysc = connect(sys1,...sysN,inputs,outputs)sys1,...sysN
based on signal names. The connect command interconnects the block
diagram elements by connecting input and output channels with matching names, as specified
in the InputName and OutputName properties of
sys1,...,sysN. The aggregate model sysc is a
dynamic system model with inputs and outputs specified by inputs and
outputs, respectively.
sysc = connect(sys1,...sysN,inputs,outputs,APs)AnalysisPoint at every signal location
specified in APs. Use analysis points to mark locations of interest
which are internal signals in the aggregate model. For instance, a location at which you
want to extract a loop transfer function or measure the stability margins is a location of
interest.
sysc = connect(blksys,connections,inputs,outputs)sysc out of an aggregate,
unconnected model blksys. The matrix connections
specifies how the outputs and inputs of blksys interconnect. For
index-based interconnections, inputs and outputs
are index vectors that specify which inputs and outputs of blksys are
the external inputs and outputs of sysc. This syntax can be convenient
when you do not want to assign names to all inputs and outputs of all models to connect.
However, in general, it is easier to keep track of named signals.
sysc = connect(___,opts)sysc. To create
opts, use connectOptions. You can use
opts with the input arguments of any of the previous syntaxes.
Examples
SISO Control System
Create an aggregate model of the following block diagram from r to y.
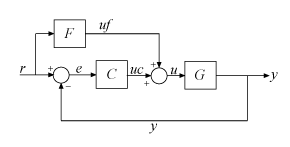
This model contains a plant G, a feedback controller C, and a feedforward controller F. Create dynamic system models representing each of these elements.
C = pid(2,1); G = zpk([],[-1,-1,-5],1); F = tf(3,[1 3]);
In preparation for building the block diagram using signal names, assign to each element the input and output names shown in the block diagram. To do so, set the InputName and OutputName properties of the elements.
C.InputName = "e"; C.OutputName = "uc"; G.InputName = "u"; G.OutputName = "y"; F.InputName = "r"; F.OutputName = "uf";
The block diagram also contains two summing junctions. One summing junction takes the difference between the reference signal r and the plant output y to compute the error signal e. The other summing junction adds the feedforward controller output uf to the feedback controller output uc to compute the plant input u. Use the sumblk command to create these summing junctions. To use sumblk, write the expression for the summing junction as a string.
S1 = sumblk("e = r - y"); S2 = sumblk("u = uc + uf");
sumblk returns a tf model object representing the sum, with the input names and output names you provide in the expression. For instance, examine the signal names of S1.
S1
S1 = From input "r" to output "e": 1 From input "y" to output "e": -1 Static gain.
You can now combine all the elements to create an aggregate model representing the response of the system in the block diagram from r to y. Provide connect with the list of elements to combine, the desired input signal of the aggregate model r, and the desired output signal y. The connect command automatically joins the elements by connecting inputs and outputs with matching names.
T = connect(G,C,F,S1,S2,"r","y"); size(T)
State-space model with 1 outputs, 1 inputs, and 5 states.
T.InputName
ans = 1x1 cell array
{'r'}
T.OutputName
ans = 1x1 cell array
{'y'}
MIMO Control System
Create the control system of the previous example, where G, C, and F are all two-input, two-output models.
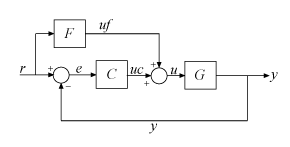
In this example, the signals r, y, e, and so on are all vector signals of two elements each. First, create the models and name their inputs and outputs.
C = [pid(2,1), 0;
0,pid(5,6)];
F = [tf(3,[1 3]), 0;
0, tf(3,[1 3])];
G = ss(-1,[1,2],[1;-1],0);Assign the input and output names.
C.InputName = "e"; C.OutputName = "uc"; G.InputName = "u"; G.OutputName = "y"; F.InputName = "r"; F.OutputName = "uf";
When you assign single names to vector-valued signals, the software automatically applies vector expansion to give each input and output channel a unique name. For instance, examine the names of the plant inputs.
G.InputName
ans = 2x1 cell
{'u(1)'}
{'u(2)'}
This example uses vector expansion for the signal names of all MIMO components of the block diagram. You can instead name the signals individually, provided that you match the names of signals you want to join. For an example that uses individually named signals for some block-diagram elements, see MIMO Control System with Fixed and Tunable Components.
Next, create the vector-valued summing junction. sumblk also automatically expands the signal names you provide in the sum expression for the vector signals, as you can verify by examining the input names of S2.
S1 = sumblk("e = r - y",2); S2 = sumblk("u = uc + uf",2); S2.InputName
ans = 4x1 cell
{'uc(1)'}
{'uc(2)'}
{'uf(1)'}
{'uf(2)'}
Combine all the elements to create an aggregate model representing the response r to y, that is, from [r(1),r(2)] to [y(1),y(2)].
T = connect(G,C,F,S1,S2,"r","y"); size(T)
State-space model with 2 outputs, 2 inputs, and 5 states.
T.InputName
ans = 2x1 cell
{'r(1)'}
{'r(2)'}
T.OutputName
ans = 2x1 cell
{'y(1)'}
{'y(2)'}
Feedback Loop with Analysis Point
Consider the following block diagram.
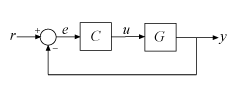
You can create a model of this closed-loop system using feedback and use the model to study the system response from r to y. Suppose that you also want to study the response of the closed-loop system to a disturbance injected at the plant input. To do so, you can use connect to build the system, inserting an analysis point at the location u.
First create plant and controller models, naming the inputs and outputs as shown in the diagram.
C = pid(2,1); C.InputName = "e"; C.OutputName = "u"; G = zpk([],[-1,-1],1); G.InputName = "u"; G.OutputName = "y";
Create a summing junction that takes the difference between the reference signal r and the plant output y to compute the error signal e.
Sum = sumblk("e = r - y");Combine C, G, and the summing junction to create the aggregate model. Use the APs input argument to connect to insert an analysis point at u.
input = "r"; output = "y"; APs = "u"; CL = connect(G,C,Sum,input,output,APs)
Generalized continuous-time state-space model with 1 outputs, 1 inputs, 3 states, and the following blocks: CONNECT_AP1: Analysis point, 1 channels, 1 occurrences. Type "ss(CL)" to see the current value and "CL.Blocks" to interact with the blocks.
This closed-loop model is a generalized state-space (genss) model containing an AnalysisPoint control design block. To see the name of the analysis point channel in CL, use getPoints.
getPoints(CL)
ans = 1x1 cell array
{'u'}
Inserting the analysis point creates a model that is equivalent to the following block diagram, where AP_u designates the AnalysisPoint block with channel name u.
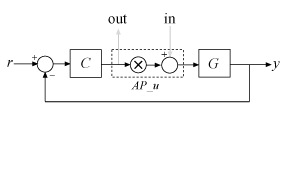
Use the analysis point to extract system responses. For example, the following commands extract the open-loop transfer at u and the closed-loop response at y to a disturbance injected at u.
L = getLoopTransfer(CL,"u",-1); CLdist = getIOTransfer(CL,"u","y");
Index-Based Interconnection
You can use index-based interconnection to connect model elements without naming all their inputs and outputs. To see how, create a model of the following feedback loop from r to y using index-based interconnection.

First, create the plant and controller, the elements of the closed-loop system.
C = pid(2,1); G = zpk([],[-1,-1,-5],1);
Use append to group the elements into a single, unconnected aggregate model.
blksys = append(C,G);
append arranges the specified models into a MIMO stacked model, as shown in the following diagram.
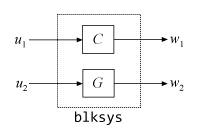
To make this stacked model equivalent to the feedback loop, connect must make the following connections:
The input of
Greceives the output ofC, orw1feeds intou2.The input of
Creceives the negative of the output ofG, or-w2feeds into-u1.
To instruct connect to make these connections, construct a matrix in which each row specifies one connection or summing junction in terms of the input vector u and output vector y of blksys.
connections = [2 1; % w1 feeds into u2 1 -2]; % -w2 feeds into u1
Finally, specify by index which inputs and outputs of blksys to use for the external inputs and outputs of the closed-loop model.
inputs = 1; % r drives u1 outputs = 2; % y is y2
You can now complete the closed-loop model.
sysc = connect(blksys,connections,inputs,outputs); step(sysc)

Combine Two Interconnected Models with Analysis Points
This example shows what to expect when combining two models created with connect, each containing analysis points.
Create a model of cascaded feedback loops using these commands.
G1 = tf([1],[1 0]); G1.u = 'OuterError'; G1.y = 'InnerCmd'; G2 = tf([1], [1 1]); G2.u = 'InnerError'; G2.y = 'ActuatorCmd'; SumOuter = sumblk('OuterError = OuterCmd - Outer'); SumInner = sumblk('InnerError = InnerCmd - Inner'); Sys1 = connect(G1,G2,SumOuter,SumInner,{'OuterCmd','Outer','Inner'},'ActuatorCmd', {'InnerError','OuterError'})
Generalized continuous-time state-space model with 1 outputs, 3 inputs, 2 states, and the following blocks: CONNECT_AP1: Analysis point, 2 channels, 1 occurrences. Type "ss(Sys1)" to see the current value and "Sys1.Blocks" to interact with the blocks.
The generalized model Sys1 contains a single analysis point block with channels InnerError and OuterError.
Alternatively, you can define inner and outer loops separately.
P1 = connect(G1,SumOuter,{'OuterCmd','Outer'},'InnerCmd','OuterError')Generalized continuous-time state-space model with 1 outputs, 2 inputs, 1 states, and the following blocks: CONNECT_AP1: Analysis point, 1 channels, 1 occurrences. Type "ss(P1)" to see the current value and "P1.Blocks" to interact with the blocks.
P2 = connect(G2,SumInner,{'InnerCmd','Inner'},'ActuatorCmd','InnerError')Generalized continuous-time state-space model with 1 outputs, 2 inputs, 1 states, and the following blocks: CONNECT_AP1: Analysis point, 1 channels, 1 occurrences. Type "ss(P2)" to see the current value and "P2.Blocks" to interact with the blocks.
Both P1 and P2 have an analysis block named CONNECT_AP1. Now combine these two models to create a model Sys2 of the cascaded loop.
Sys2 = connect(P1,P2,{'OuterCmd','Outer','Inner'},'ActuatorCmd')Generalized continuous-time state-space model with 1 outputs, 3 inputs, 2 states, and the following blocks: CONNECT_AP1: Analysis point, 1 channels, 1 occurrences. CONNECT_AP2: Analysis point, 1 channels, 1 occurrences. Type "ss(Sys2)" to see the current value and "Sys2.Blocks" to interact with the blocks.
This model contains two separate analysis point blocks with names CONNECT_AP1 and CONNECT_AP2. The AnalysisPoint blocks of P1 and P2 are automatically renamed to avoid conflict.
Input Arguments
sys — Dynamic system model or other element to interconnect
LTI model object | control design block | AnalysisPoint block | identified model | sparse model object | time-varying model object | parameter-varying model object | time-varying model object
Dynamic system model or other block-diagram element to interconnect, specified as:
Any numeric LTI model object, such as a
tf,zpk,ss,frd, orpidmodel object.A generalized or uncertain LTI model, such as a
genss,genfrd,uss(Robust Control Toolbox), andufrd(Robust Control Toolbox) model.A control design block representing a tunable or uncertain block-diagram element, such as a
tunablePID,tunableSS,tunableGain,tunableTF,tunableSurface,ultidyn(Robust Control Toolbox), orumargin(Robust Control Toolbox) block.An
AnalysisPointblock representing a location in the block diagram at which you want to extract system responses.A summing junction that you create using
sumblk.An identified LTI model, such as an
idtf(System Identification Toolbox),idss(System Identification Toolbox), oridproc(System Identification Toolbox) model.A sparse model, represented by a
sparssormechssmodel object.A time-varying or parameter-varying model, represented by an
ltvssorlpvssmodel object.
inputs — Inputs of combined model
character vector | cell array of character vectors | string | string vector | positive integer | vector of positive integers
Inputs of the combined model, specified as a character vector, cell array of character vectors, string, string vector, positive integer, or vector of positive integers.
For name-based interconnection, provide a character vector, string, cell array of character vectors, or string array listing one or more signals that appear in the
InputNameorOutputNameproperty of one or more of the block diagram elementssys1,...sysN. For example,{'ref','dist','noise'}.For index-based interconnection, provide a positive integer or vector of positive integers specifying the index or indices of the inputs of
blksysthat you want to be inputs of the aggregate model.
outputs — Outputs of combined model
character vector | cell array of character vectors | string | string vector | positive integer | vector of positive integers
Outputs of the combined model, specified as a character vector, cell array of character vectors, string, string vector, positive integer, or vector of positive integers.
For name-based interconnection, provide a character vector, string, cell array of character vectors, or string array listing one or more signals that appear in the
InputNameorOutputNameproperty of one or more of the block diagram elementssys1,...sysN. For example,{'ref','dist','noise'}.For index-based interconnection, provide a positive integer or vector of positive integers specifying the index or indices of the inputs of
blksysthat you want to be outputs of the aggregate model.
APs — Locations of interest in the combined model
character vector | cell array of character vectors | string | string vector
Locations (internal signals) of interest in the aggregate model, specified as a
character vector or cell array of character vectors, such as 'X' or
{'AP1','AP2'}, or a string or string vector. The resulting model
contains an analysis point at each such location. (See AnalysisPoint). Each location in APs must correspond
to an entry in the InputName or OutputName
property of one or more of the block diagram elements
sys1,...sysN.
blksys — Unconnected aggregate model
dynamic system model
Unconnected aggregate model, specified as a dynamic system model that you create
with append. Use blksys
for index-based interconnection. The append command stacks the
inputs and outputs of the elements of your block diagram without making any
interconnections between their inputs and outputs. For example, if your block diagram
contains dynamic system models C, G, and
S, create blksys with the following
command:
blksys = append(C,G,S)
Then, specify the interconnections between the inputs and outputs of
blksys using the connections argument. For
an example, see Index-Based Interconnection.
connections — Connections and summing junctions
matrix
Connections and summing junctions of the block diagram, specified as a matrix. Use
connection for index-based interconnection. Each row of
connections specifies one connection or summing junction in terms
of the input vector u and output vector w of the
unconnected aggregate model blksys. For example, the row
[3 2 0 0]
specifies that w(2), the second output of
blksys, connects into u(3), the third input of
blksys. The row
[7 2 -15 6]
indicates that the sum w(2) - w(15) + w(6) feeds into
u(7), the seventh input of blksys.
If you do not specify any connection for a particular input or output,
connect omits that input or output from the aggregate
model.
opts — Additional options for interconnection
connectOptions options set
Additional options for interconnection, specified as an options set that you create
with connectOptions.
By default, the connect command discards states that do not
contribute to the dynamics in the path between the inputs and outputs of the
interconnected system. Use connectOptions to retain these states in
the interconnected model. This option can be useful, for example, when you want to
compute the interconnected system response from known initial state values of the
components.
Output Arguments
sysc — Interconnected system
state-space model | frequency-response data model
Interconnected system, returned as either a state-space model or frequency-response model. The type of model returned depends on the input models. For example:
Interconnecting numeric LTI models (other than
frdmodels) returns anssmodel.Interconnecting a numeric LTI model with a Control Design Block returns a generalized state-space model. For instance, interconnecting a
tfmodel with atunablePIDControl Design Block returns agenssmodel.Interconnecting a numeric LTI model with a sparse model returns a sparse model.
Interconnecting any model with a frequency-response data model returns a frequency response data model.
By default, connect automatically discards states that do not
contribute to the I/O transfer function from the specified inputs to the specified
outputs of the interconnected model. To retain the unconnected states, set the
Simplify option of connectOptions to false. For example:
opt = connectOptions('Simplify',false); sysc = connect(sys1,sys2,sys3,'r','y',opt);
Version History
Introduced before R2006aR2024a: Support for connecting multiple interconnected systems with analysis points
You can specify analysis points when modeling block diagrams with connect. When combining the resulting models with any interconnection
function, the software automatically renames these analysis point blocks to avoid conflict.
Before R2024a, such combinations were not possible.
For an example, see Combine Two Interconnected Models with Analysis Points.
R2015a: Syntax for inserting analysis points
You can now specify analysis point locations using the APs input
argument, using the following syntax.
sysc = connect(sys1,sys2,...,sysN,inputs,outputs,APs)
For an example, see Feedback Loop with Analysis Point.
R2013b: Option to retain unconnected states
By default, the connect command discards states that do not
contribute to the dynamics in the path between the inputs and outputs of the interconnected
system. You can now use the opts input argument to retain such
unconnected states. This option can be useful, for example, when you want to compute the
interconnected system response from known initial state values of the components. Create
opts with connectOptions.
R2013b: connect returns state-space or frequency response data model
connect always returns a state-space model, such as an
ss, genss, or uss model,
unless one or more of the input models is a frequency response data model. In that case,
connect returns a frequency response data model, such as an
frd or genfrd model. In previous releases,
connect returned a tf or
zpk model when all input models were tf or
zpk models.
MATLAB Command
You clicked a link that corresponds to this MATLAB command:
Run the command by entering it in the MATLAB Command Window. Web browsers do not support MATLAB commands.

Select a Web Site
Choose a web site to get translated content where available and see local events and offers. Based on your location, we recommend that you select: .
You can also select a web site from the following list
How to Get Best Site Performance
Select the China site (in Chinese or English) for best site performance. Other bat365 country sites are not optimized for visits from your location.
Americas
- América Latina (Español)
- Canada (English)
- United States (English)
Europe
- Belgium (English)
- Denmark (English)
- Deutschland (Deutsch)
- España (Español)
- Finland (English)
- France (Français)
- Ireland (English)
- Italia (Italiano)
- Luxembourg (English)
- Netherlands (English)
- Norway (English)
- Österreich (Deutsch)
- Portugal (English)
- Sweden (English)
- Switzerland
- United Kingdom (English)
Asia Pacific
- Australia (English)
- India (English)
- New Zealand (English)
- 中国
- 日本Japanese (日本語)
- 한국Korean (한국어)